New Movie as quicktime:
Movie as animated GIF:
Movie as quicktime:
Movie as AVI:
(Although we cannot submit a movie, we can submit URL for movie :-))
Figure 1. Cross sections illustrating possible vertical variation in displacement (d) across strike-slip fault systems with total displacement D. The two left panels (A and C) have distributed deformation in the mantle, the right panels (B and D) have highly localized deformation in the mantle. Areas with predominantly subhorizontal schistosity (and presumably subhorizonal planar seismic anisotropy) indicated by horizontal ruling; in all cases, for high strains lineations in strained rocks will be normal to the section. Thinner lines follow surfaces of constant displacement. (A) A thick (thickness t) and wide (width w) zone in the lower crust connects a surface fault to distributed deformation in the mantle. (B) Lithospheric deformation localized on a vertical plane; note the potential Moho offset. (C) Similar to (A) but with a very thin (t << w) zone connecting upper crust to mantle deformation. Note the more intense planar anisotropy. Although shown at the base of the crust, it is possible a zone such as this could be higher in the crust. As t goes to zero, this would be a decollement. (D). Several surface faults above a single fault in the upper mantle. Note a central zone of subvertical anisotropy lies between zones of subhorizontal anisotropy. Multiple faults over distributed mantle deformation, as in (A), could be viewed as diagram A repeated several times or the part of (D) above the "subvertical anisotropy". (E, bottom) Four perspective images of the progressive deformation of an initially rectangular block of rigid upper crust (red), ductile lower crust (yellow) and unifromly-straining upper mantle (red) illustrating the kind of deformation shown in (A). A movie of this deformation can be viewed at http://cires.colorado.edu/people/jones.craig/NewZealand/NewZealandFigs.html.(which is this page)
Figure 2. Example of azimuthal variations in S waves formed by conversions at an interface separating an isotropic layer from an anisotropic one. Amplitudes have been corrected for incidence angle. The amplitude of the direct P wave (for reference) is above the amplitudes on the radial receiver function of a mid-crustal negative converter (MCN) interpreted as the top of a body of sheared crust, a lower crustal postive (LCP) interpreted as the base of the sheared crust, and the Moho. Note the absence of a two-theta variation on the Moho conversion (the 1-theta pattern is consistent with dip on the Moho also seen in differences in Ps-P times over backazimuth). Also note the factor of 3 variation in the MCN amplitude compared with the ~50% variation in the Moho Ps amplitude. Gray boxes are from the SW backazimuth where the Moho Ps is locally extinguished, probably by melt associated with a Holocene volcanic field, and these points were excluded when making the best fit curves.
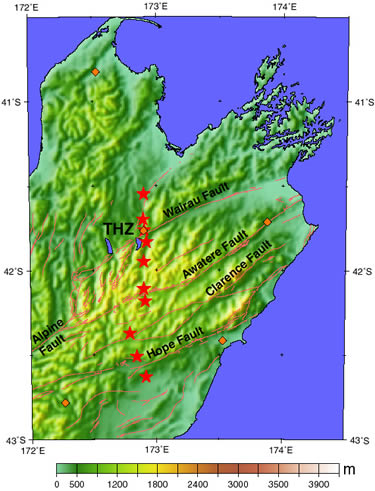
Figure 3. Map of the Marlborough region showing the major faults, shaded topography, and stations. Slip rates on the faults are summarized in Table 1.
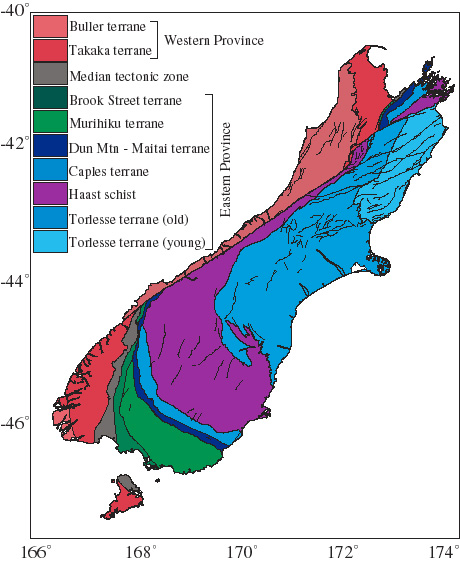
Figure 4. Terrane map of the South Island, showing the offset of the Alpine fault system.
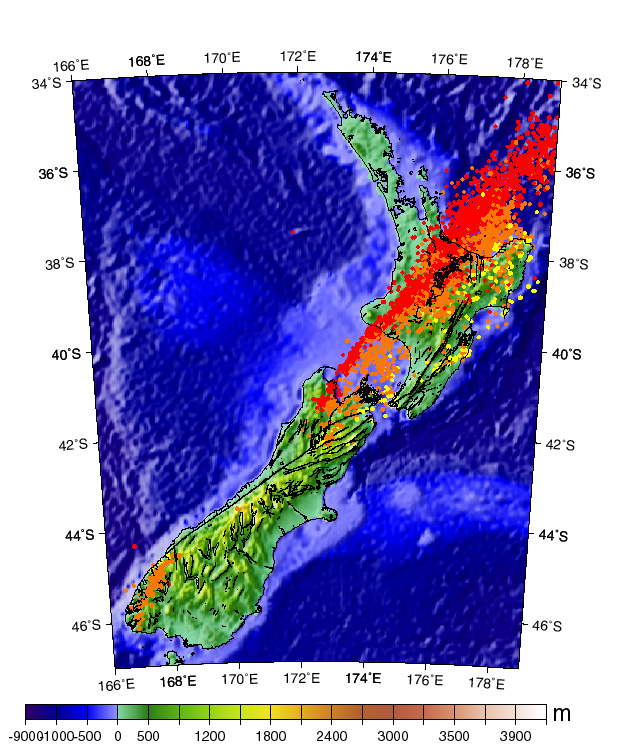
Figure 5. (A) Plot of earthquakes M>=3.5 beneath the northern end of the South Island and the southern end of the North Island from 1/1/90 to 11/20/94 below 50 km. Yellow: 50-60 km, Orange, 60-150 km, Red, below 150 km depth.
(B) Polar plot of vectors to earthquakes M>=3.0 depth >= 50 km from 1/1/94 to 6/30/94 from 172.8° E 41.5 ° S.
Figure 6. Shear-wave splitting polarizations at THZ (Fig. 3) from Audoine et al. [1999]. (Left) split time (s) vs. earthquake depth with teleseismic SKS results shown at top right, (right) rose diagram of fast direction of SV propogation at THZ.
Figure 7. Synthetic seismograms generated over a flat interface (here, a 30 km deep Moho), modified from Clouser and Langston [1995]. The receiver function, which is the deconvolution of the vertical from the radial, will not show the phases with a final P leg (e.g., PpPmp; these are lumped with the zero-lag response).
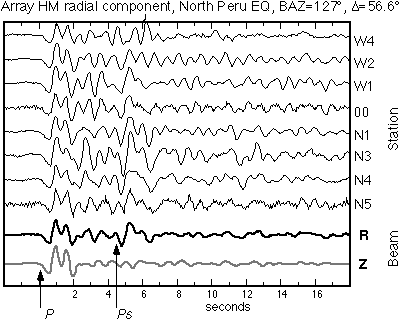
Figure 8. Example of the improvement in signal quality through stacking of individual radial short-period seismometers (top traces) from a M5.8 teleseism into beams (bottom two traces), from the 1993 SSCD experiment (Jones and Phinney, 1998).
Figure 9. (a) Discontinuity image from Snake River Plain PASSCAL Experiment data (Dueker and Sheehan, 1997). Each trace shown represents a common midpoint stack (CMP) of all receiver functions within a 75 km wide bin stacked along appropriate normal moveout curves and with lateral heterogeneity corrections applied before the stack. Positive and negative polarity energy is plotted in black and gray, respectively. (b) migrated snake river plain discontinuity image (Sheehan et al., 1999). Migration is performed by backprojection, similar to Kirchoff migration used in traditional reflection seismology.
Figure 10. Synthetic experiments with migration seismic processing of receiver functions (Sheehan et al., 1999). (A) Model containing undulating mid-crustal discontinuity, Moho with 8 km offset, and 45 degree dipping truncated discontinuity at 65 to 80 km depth. . Synthetic seismograms are generated using the same geometry of sources and receivers as in our proposed Marlborough Fault Zone experiment. (B) Common midpoint stack of synthetic receiver functions. "Clumpiness" is due to station geometry with tight arrays of six stations (only using N-S arm of each array for this synthetic experiment) 500 m apart with 7 km offset between subsequent arrays. CMP stacking with 2 km uniform station spacing (not shown) does not have the 'clumpiness' seen here but exhibits classic diffraction and focusing artifacts, having particular difficulty with the shallow undulating discontinuity and the truncated tip of the dipping discontinuity. (C) Backprojection migration of synthetic receiver functions. The starting model is recovered well, including the sharp offset in the Moho and the steeply dipping truncated discontinuity. Good results are obtained both with the proposed New Zealand array geometry (shown here) and with uniform station spacing (not shown).
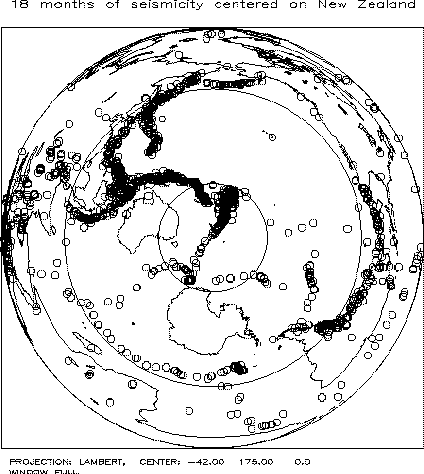
Map of 18 months of seismicity (mb>4.5) centered on New Zealand, illustrating distribution of (teleseismic) seismicity and sample seismic rates. Inner circles represent thirty and ninety degree epicentral distance (most useful range for teleseismic receiver functions). Abundant seismicity at a wide range of distances (ray parameter) and azimuth is apparent. Lambert projection.
Cartoon of the influence of converter dip, anisotropy, and scattering on radial and transverse components of a receiver function, from Jones and Phinney (1998).
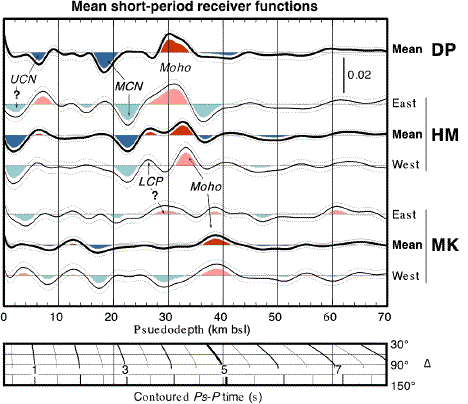
Mean receiver functions from the 1993 SSCD experiment, from Jones and Phinney (1998).